The Physics of Racing,
Part 26: The Driving Wheel, Chapter I
Brian Beckman, PhD
©Copyright July 2001
Imagine 400 ft-lbs of torque measured on a chassis dynamometer like a DynoJet
(see references at the end). This is a very nice number to have in any car,
street or racing. In dyno-speak, however, the interpretation of this number is a
little tricky.
To start a dyno session, you strap your car down with the driving wheels over
a big, heavy drum, then you run up the gears gently and smoothly until you're in
fourth at the lowest usable engine RPM, then you floor it, let the engine run
all the way to redline, then shut down. The dyno continuously measures the time
and speed of the drum and the engine RPM through a little remote radio receiver
that picks up spark-plug noise. The only things resisting the motion of the
driving wheels are the inertia of the driveline in the car and the inertia of
the drum. The dyno 'knows' the latter, but not the former. Without these
inertias loading the engine, it would run up very quickly and probably blow up.
Test-stand dynos, which run engines out of the car, load them in different ways
to prevent them from free running to annihilation. Some systems use water
resistance, others use electromagnetic in any case, the resistance must be easy
to calibrate and measure. We are only concerned with chassis dynos in this
article, however.
What is the equation of motion for the car + dyno system? It is a simple
variation on the theme of the old, familiar second law of Newton. For linear
motion, that law has the form
F = ma, where F is the net
force on an object, m is its mass, and a is its
acceleration, or time rate of change of velocity.
For rotational motion, like that of the driveline and dyno, Newton's second
law takes the form 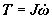 where T is the net torque on an object,
J is its moment of inertia, and
where T is the net torque on an object,
J is its moment of inertia, and 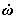 is its angular acceleration, or time rate
of change of angular velocity. The purpose of this instalment of
the Physics of Racing is to explain everything here and to run a few
numbers.
is its angular acceleration, or time rate
of change of angular velocity. The purpose of this instalment of
the Physics of Racing is to explain everything here and to run a few
numbers.
To get the rotational equation of motion, we assume that the dyno drum is
strong enough that it will never fly apart, no matter how fast it spins. We
model it, therefore, as a bunch of point masses held to the centre of rotation
by infinitely strong, massless cables. With enough point masses, we can
approximate the smooth (but grippy) surface of the dyno drum as closely as we
would like.
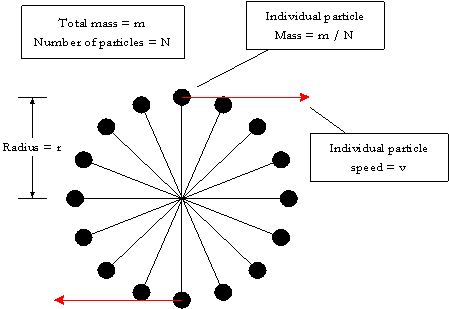
Assume each particle receives a force of F / N in the
tangential direction. Tangential, of course, means the same as circumferential
or longitudinal, as clarified in recent instalments about slip and grip of
tyres. So, each particle accelerates according to
F / N = ma / N. The N
cancels out, leaving a = F / m. Now,
a is the rate of change of the velocity, and the velocity is
defined as 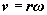 , where r is the constant radius of the
circle and
, where r is the constant radius of the
circle and  is the angular velocity in radians per
second. The circumference of the circle is
is the angular velocity in radians per
second. The circumference of the circle is 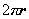 , by definition, so a drum of 3-foot radius has a
circumference of about 6.28 x 3 = 18.8 feet. At 60 RPM, which is one rev per
second, each particle goes 18.8 feet per second, which is about 15 x 18.8/22 =
12.8 mph. RPM is one measurement of angular velocity, but it's more convenient
to measure it such that
, by definition, so a drum of 3-foot radius has a
circumference of about 6.28 x 3 = 18.8 feet. At 60 RPM, which is one rev per
second, each particle goes 18.8 feet per second, which is about 15 x 18.8/22 =
12.8 mph. RPM is one measurement of angular velocity, but it's more convenient
to measure it such that 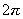 angular units go by every second. Such units save us
from having to track factors of
angular units go by every second. Such units save us
from having to track factors of 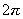 all over the math.
So, there are
all over the math.
So, there are 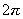 radians per revolution, and the equation
radians per revolution, and the equation 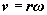 is seen as a general expression of the example that
is seen as a general expression of the example that
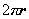 x revolutions per second = 18.8 feet per
second = velocity.
x revolutions per second = 18.8 feet per
second = velocity.
Since r is constant, it has no rate of change. Only  has one, measured in radians per second per
second, or radians per second squared, or
has one, measured in radians per second per
second, or radians per second squared, or 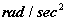 , and denoted with
an overdot:
, and denoted with
an overdot: 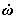 . The equation of motion, so far, looks like
. The equation of motion, so far, looks like 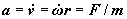 . Now, we know that torque is just force
times the lever arm over which the force is applied. So, a force of
F at the surface of the drum translates into a torque of T =
Fr applied to the shaft-or by the shaft, depending on point of view. So
we write
. Now, we know that torque is just force
times the lever arm over which the force is applied. So, a force of
F at the surface of the drum translates into a torque of T =
Fr applied to the shaft-or by the shaft, depending on point of view. So
we write 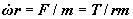 , which we can rearrange to
, which we can rearrange to 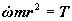 . We can make this resemble the linear form of
Newton's law if we define
. We can make this resemble the linear form of
Newton's law if we define 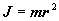 , the moment of inertia of the drum, yielding <
, the moment of inertia of the drum, yielding <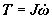 , which looks just like>F = ma if
we analogise as follows:
, which looks just like>F = ma if
we analogise as follows: 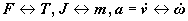 .
.
This value for J only works for this particular model of
the drum, with all the mass elements at distance r from the
centre. Suffice it to say that a moment of inertia for any other model of the
drum could be computed in like manner. It turns out that the moment of inertia
of a solid cylindrical drum is half as much, namely 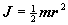 . Moments of inertia for common shapes can be looked up
all over the place, for instance at http://www.physics.uoguelph.ca/tutorials/torque/Q.torque.inertia.html.
. Moments of inertia for common shapes can be looked up
all over the place, for instance at http://www.physics.uoguelph.ca/tutorials/torque/Q.torque.inertia.html.
So, now, the dyno has a known, fixed value for J, and it
measures 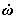 very accurately. This enables it to calculate trivially
just how much torque is being applied by the driving wheels of the car to the
drum. But it does not know the moment of inertia of the driveline of the
car, let alone the radius of the wheels, the gear selected by the driver, the
final-drive ratio in the differential, and so on. In other words, it knows
nothing about the driveline other than engine RPM.
very accurately. This enables it to calculate trivially
just how much torque is being applied by the driving wheels of the car to the
drum. But it does not know the moment of inertia of the driveline of the
car, let alone the radius of the wheels, the gear selected by the driver, the
final-drive ratio in the differential, and so on. In other words, it knows
nothing about the driveline other than engine RPM.
Everyone knows that the transmission and final-drive on a car multiply
the engine torque. The torque at the driving wheels is almost always much larger
than the flywheel torque, and it's larger in lower gears than in higher gears.
So, if you run up the dyno in third gear, it will accelerate faster than if you
run it up in fourth gear. Yet, the dyno reports will be comparable. Somehow,
without knowing any details about the car, not even drastic things like gear
choice, the dyno can figure out flywheel torque. Well, yes and no.
It turns out that all the dyno needs to know is engine RPM. It does not
matter whether the dyno is run up quickly with a relatively large drive-wheel
torque (DWT) or run up slowly with a relatively small
DWT. Furthermore, the radius of the driving wheels and tyres also
does not matter. Here's why.
Wheel RPM is directly proportional to drum RPM, assuming the longitudinal
slip of the tyres is within a small range. The reason is that at the point of
contact, the drum and wheel have the same circumferential (longitudinal,
tangential) speed, so  .
Let's write
.
Let's write 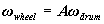 ,
where
,
where 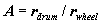 . Engine RPM is related to wheel RPM by a factor that
depends on the final-drive gear ratio f and the selected gear
ratio
. Engine RPM is related to wheel RPM by a factor that
depends on the final-drive gear ratio f and the selected gear
ratio  . We write
. We write 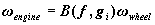 .
Usually, engine RPM is much larger than wheel RPM, so we can expect
.
Usually, engine RPM is much larger than wheel RPM, so we can expect 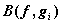 to be larger than 1 most of the time. So, we get
to be larger than 1 most of the time. So, we get
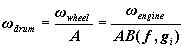
We also know that, by Newton's Third Law, that the force applied to the drum
by the tyre is the same as the force applied to the tyre by the drum. Therefore
the torques applied are in proportion to the radii of the wheel + tyre and the
drum, namely that
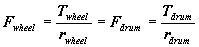
or 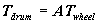 . Recalling that the transmission gear and final drive
multiply engine torque, we also know that
. Recalling that the transmission gear and final drive
multiply engine torque, we also know that 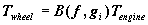 ,
so
,
so 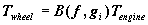 .
But we already know
.
But we already know 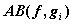 : it's the ratio of the RPMs,
so
: it's the ratio of the RPMs,
so 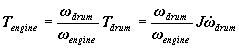 , or, more usefully,
, or, more usefully,
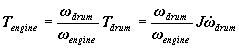
Every term on the right-hand side of this equation is measured or known by
the dyno, so we can measure engine torque independently of car details! We can
even plot 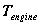 versus
versus  , effectively taking the run-up time and the drum data
out of the report.
, effectively taking the run-up time and the drum data
out of the report.
Almost. There is a small gotcha. The engine applies torque indirectly to the
drum, spinning it up. But the engine is also spinning up the clutch,
transmission, drive shaft, differential, axles, and wheels, which, all together,
have an unknown moment of inertia that varies from car-to-car, though it's
usually considerably smaller than J, the moment of inertia of the
drum. But, in the equations of motion, above, we have not accounted for them.
More properly, we should write
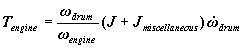
This doesn't help us much because we don't know 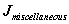 , so we pull a fast one and rearrange the equation:
, so we pull a fast one and rearrange the equation:

This is why chassis dyno numbers are always lower than test-stand dyno
numbers for the same engine. The chassis dyno measures 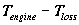 ,
and the test-stand measures
,
and the test-stand measures 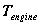 . Of course, those trying to sell engines often report
the best-sounding numbers: the test-stand numbers. So, don't be disappointed
when you take your hot, new engine to the chassis dyno after installation and
get numbers 15% to 20% lower than the advertised 'at the crankshaft' numbers in
the brochure. It's to be expected. Typically, however, you simply do not
know
. Of course, those trying to sell engines often report
the best-sounding numbers: the test-stand numbers. So, don't be disappointed
when you take your hot, new engine to the chassis dyno after installation and
get numbers 15% to 20% lower than the advertised 'at the crankshaft' numbers in
the brochure. It's to be expected. Typically, however, you simply do not
know
 : it's a number you take on faith.
: it's a number you take on faith.
Let's run a quick sample. The following numbers are pulled out of thin air,
so don't hang me on them. Suppose the drum has 3-foot radius, is solid, and
weighs 6,400 lbs, which is about 200 slugs (remember slugs? One slug of mass
weighs about 32 pounds at the Earth's surface). So, the moment of inertia of the
drum is about 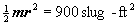 . Let's say that the engine takes about 15 seconds to run
from 1,500 RPM to 6,000 RPM in fourth gear, with a time profile like the
following:
. Let's say that the engine takes about 15 seconds to run
from 1,500 RPM to 6,000 RPM in fourth gear, with a time profile like the
following:
| t | e RPM | V MPH | v FPS | drum  | drum 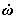 | drum RPM | RPM ratio | Torque |
| 0 | 1,500 | 35 | 51.33 | 17.11 | 0.00 | 163.40 | 0.1089 | 0.00 |
| 1 | 1,800 | 42 | 61.60 | 20.53 | 3.42 | 196.08 | 0.1089 | 335.51 |
| 2 | 2,100 | 49 | 71.87 | 23.96 | 3.42 | 228.76 | 0.1089 | 335.51 |
| 3 | 2,400 | 56 | 82.13 | 27.38 | 3.42 | 261.44 | 0.1089 | 335.51 |
| 4 | 2,700 | 63 | 92.40 | 30.80 | 3.42 | 294.12 | 0.1089 | 335.51 |
| 5 | 3,000 | 70 | 102.67 | 34.22 | 3.42 | 326.80 | 0.1089 | 335.51 |
| 6 | 3,300 | 77 | 112.93 | 37.64 | 3.42 | 359.48 | 0.1089 | 335.51 |
| 7 | 3,600 | 84 | 123.20 | 41.07 | 3.42 | 392.16 | 0.1089 | 335.51 |
| 8 | 3,900 | 91 | 133.47 | 44.49 | 3.42 | 424.84 | 0.1089 | 335.51 |
| 9 | 4,200 | 98 | 143.73 | 47.91 | 3.42 | 457.52 | 0.1089 | 335.51 |
| 10 | 4,500 | 105 | 154.00 | 51.33 | 3.42 | 490.20 | 0.1089 | 335.51 |
| 11 | 4,800 | 112 | 164.27 | 54.76 | 3.42 | 522.88 | 0.1089 | 335.51 |
| 12 | 5,100 | 119 | 174.53 | 58.18 | 3.42 | 555.56 | 0.1089 | 335.51 |
| 13 | 5,400 | 126 | 184.80 | 61.60 | 3.42 | 588.24 | 0.1089 | 335.51 |
| 14 | 5,700 | 133 | 195.07 | 65.02 | 3.42 | 620.92 | 0.1089 | 335.51 |
| 15 | 6,000 | 140 | 205.33 | 68.44 | 3.42 | 653.60 | 0.1089 | 335.51 |
The " v MPH" column is just a straight linear ramp from 35 MPH to 140 MPH,
which are approximately right in my Corvette. The " v FPS" column is just 22 / 15
the v MPH. The drum  is in radians per second and is just v FPS divided
by 3 ft, the drum radius. The drum
is in radians per second and is just v FPS divided
by 3 ft, the drum radius. The drum 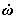 is just the stepwise difference of the drum
is just the stepwise difference of the drum
 numbers. It's constant, as we would
expect from a run-up of the dyno at constant acceleration. The drum RPM is
numbers. It's constant, as we would
expect from a run-up of the dyno at constant acceleration. The drum RPM is 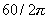 times the drum
times the drum  . The RPM ratio is just drum RPM divided by engine
RPM, and it must be strictly constant, so this is a nice sanity check on our
math. Finally, the torque column is the RPM ratio times
J = 900 slug - ft2 times drum
. The RPM ratio is just drum RPM divided by engine
RPM, and it must be strictly constant, so this is a nice sanity check on our
math. Finally, the torque column is the RPM ratio times
J = 900 slug - ft2 times drum
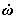 . We see a constant torque output of about
335 ft-lbs. Not bad. It implies a test-stand number of between 394 and 418,
corresponding to 15% and 20% driveline loss, respectively. Looks like we nailed
it without 'cooking the books' too badly. Of course, we have a totally flat
torque curve in this little sample, but that's only because we have a completely
smooth ramp-up of velocity.
. We see a constant torque output of about
335 ft-lbs. Not bad. It implies a test-stand number of between 394 and 418,
corresponding to 15% and 20% driveline loss, respectively. Looks like we nailed
it without 'cooking the books' too badly. Of course, we have a totally flat
torque curve in this little sample, but that's only because we have a completely
smooth ramp-up of velocity.
Dyno reports often will be labelled 'Rear-wheel torque' (RWT)
or, less prejudicially, 'drive-wheel torque' (DWT) to remind the
user that there is an unknown component to the measurement. These are well
intentioned misnomers: do not be mislead! What they mean is 'engine
torque as if the engine were connected to the drive wheels by a massless
driveline', or 'engine torque as measured at the drive wheels with an unknown
but relatively small inertial loss component'. It should be clear from the above
that the actual drive-wheel torque cannot be measured without knowing
A, the ratio of the drum radius to the wheel + tyre radius. It's
slightly ironic that an attempt to clear up the confusion risks introducing more
confusion.
In the next instalment, we relate the equations of motion for the driving
wheel to the longitudinal magic formula to compute reaction forces and get
equations of motion for the whole car.
References:
http://www.c5-corvette.com/DynoJet_Theroy.htm
[sic]
http://www.mustangdyne.com/pdfs/7K%20manualv238.pdf
http://www.revsearch.com/dynamometer/torque_vs_horsepower.html
Attachments:
I've included the little spreadsheet I used to simulate the dyno run. It can
be downloaded here.
ERRATA:
* Part 14, yet again, the numbers for frequency are
actually in radians per second, not in cycles per second. There are 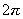 cycles per radian, so the 4 Hz natural
suspension frequency I calculated and then tried to rationalize was really 4 /
6.28 Hz, which is quite reasonable and not requiring any rationalization. Oh,
what tangled webs we weave…
cycles per radian, so the 4 Hz natural
suspension frequency I calculated and then tried to rationalize was really 4 /
6.28 Hz, which is quite reasonable and not requiring any rationalization. Oh,
what tangled webs we weave…
* Physical interpretations of slip on page 2 of part 24: " Car (hub) moving
forward, CP moving slowly forward w.r.t. ground, resisting car motion." Should
be " Car (hub) moving forward, CP moving slowly forward w.r.t. HUB,
resisting car motion."
* Part 21, in the back-of-the-envelope numerical calculation just before the
3-D plot at the end of the paper, I correctly calculated
tan-1(0.8222) = 0.688, but then incorrectly calculated
tan-1(SB) - SB as -0.266. Of course, it's
0.688 - 0.822 = -0.134. One of the hazards of doing math in one's
head all the time is the occasional slip up. Normally, I check results with a
calculator just to be really sure, but some are so trivial it just seems
unnecessary. Naturally, those are the ones that bite me.
More info
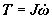 where T is the net torque on an object,
J is its moment of inertia, and
where T is the net torque on an object,
J is its moment of inertia, and 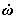 is its angular acceleration, or time rate
of change of angular velocity. The purpose of this instalment of
the Physics of Racing is to explain everything here and to run a few
numbers.
is its angular acceleration, or time rate
of change of angular velocity. The purpose of this instalment of
the Physics of Racing is to explain everything here and to run a few
numbers.
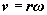 , where r is the constant radius of the
circle and
, where r is the constant radius of the
circle and  is the angular velocity in radians per
second. The circumference of the circle is
is the angular velocity in radians per
second. The circumference of the circle is 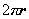 , by definition, so a drum of 3-foot radius has a
circumference of about 6.28 x 3 = 18.8 feet. At 60 RPM, which is one rev per
second, each particle goes 18.8 feet per second, which is about 15 x 18.8/22 =
12.8 mph. RPM is one measurement of angular velocity, but it's more convenient
to measure it such that
, by definition, so a drum of 3-foot radius has a
circumference of about 6.28 x 3 = 18.8 feet. At 60 RPM, which is one rev per
second, each particle goes 18.8 feet per second, which is about 15 x 18.8/22 =
12.8 mph. RPM is one measurement of angular velocity, but it's more convenient
to measure it such that 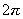 angular units go by every second. Such units save us
from having to track factors of
angular units go by every second. Such units save us
from having to track factors of 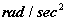 , and denoted with
an overdot:
, and denoted with
an overdot: 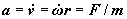 . Now, we know that torque is just force
times the lever arm over which the force is applied. So, a force of
F at the surface of the drum translates into a torque of T =
Fr applied to the shaft-or by the shaft, depending on point of view. So
we write
. Now, we know that torque is just force
times the lever arm over which the force is applied. So, a force of
F at the surface of the drum translates into a torque of T =
Fr applied to the shaft-or by the shaft, depending on point of view. So
we write 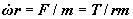 , which we can rearrange to
, which we can rearrange to 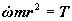 . We can make this resemble the linear form of
Newton's law if we define
. We can make this resemble the linear form of
Newton's law if we define 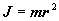 , the moment of inertia of the drum, yielding <
, the moment of inertia of the drum, yielding <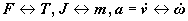 .
.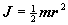 . Moments of inertia for common shapes can be looked up
all over the place, for instance at
. Moments of inertia for common shapes can be looked up
all over the place, for instance at  .
Let's write
.
Let's write 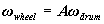 ,
where
,
where 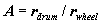 . Engine RPM is related to wheel RPM by a factor that
depends on the final-drive gear ratio f and the selected gear
ratio
. Engine RPM is related to wheel RPM by a factor that
depends on the final-drive gear ratio f and the selected gear
ratio  . We write
. We write 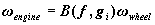 .
Usually, engine RPM is much larger than wheel RPM, so we can expect
.
Usually, engine RPM is much larger than wheel RPM, so we can expect 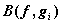 to be larger than 1 most of the time. So, we get
to be larger than 1 most of the time. So, we get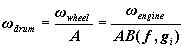
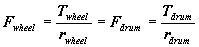
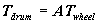 . Recalling that the transmission gear and final drive
multiply engine torque, we also know that
. Recalling that the transmission gear and final drive
multiply engine torque, we also know that 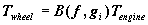 ,
so
,
so 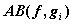 : it's the ratio of the RPMs,
so
: it's the ratio of the RPMs,
so 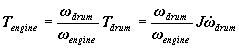 , or, more usefully,
, or, more usefully,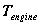 versus
versus  , effectively taking the run-up time and the drum data
out of the report.
, effectively taking the run-up time and the drum data
out of the report.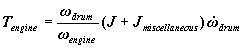
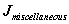 , so we pull a fast one and rearrange the equation:
, so we pull a fast one and rearrange the equation:
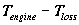 ,
and the test-stand measures
,
and the test-stand measures  : it's a number you take on faith.
: it's a number you take on faith.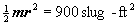 . Let's say that the engine takes about 15 seconds to run
from 1,500 RPM to 6,000 RPM in fourth gear, with a time profile like the
following:
. Let's say that the engine takes about 15 seconds to run
from 1,500 RPM to 6,000 RPM in fourth gear, with a time profile like the
following: times the drum
times the drum